Be sure to check back often, because assignments may change!
(Last modified: Friday, October 21, 2011, 10:22 AM )

All section and page numbers refer to sections from Calculus: Early Transcendental Functions, 3rd Edition, by Smith and Minton.

I'll use Maple syntax for some of the mathematical notation on this page. (Paying attention to how I type various expressions is a good way to absorb Maple notation). I will not use it when I think it will make the questions too difficult to read.

Due Monday 10/3 at 8am

Section 2.5: The Chain Rule

To read: Through Example 2.5.4. In Example 2.5.3, pay particular attention to how rewriting h(x) in various ways allows you to think of different approaches to differentiating the function.

Reading questions:

1. When do you use the chain rule to differentiate?

Due Wednesday 10/5 at 8am

Project 1

To read: Read through the entire letter requesting your assistance before class Wednesday. Make sure you understand any words you don't know the definitions of, and that you understand what you're being asked to do.

No Reading Questions Today

Reminders:

• Bring remaining questions on PS 5 to class.
• Remember to put a star next to the primary author's name on the final version of PS 5.
• I am giving you more time on the project than I ordinarily would because of Fall Break.

Due Friday 10/7 at 8am

Section 2.6: Derivatives of Trigonometric Functions
Section 2.7: Derivatives of Exponential and Logarithmic Functions (for Thursday)

To read: All. In Section 2.6, pay particular attention to the clever steps in the proof of Lemma 2.6.4, and to the use of trigonometric identities and factoring in the proof of Theorem 2.6.1. In Section 2.7, pay particular attention to the way the chain rule comes into play wiht exponential functions in Examples 2.7.2 and 2.7.3. Also, just be aware that we will be seeing a slightly different proof of Theorem 2.7.3.

Reading questions:

1. What is lim _{h → 0} ^sin(h)⁄_h?
2. What is lim _{h → 0} ^(1-cos(h))⁄_h?
3. Why are the limits in the first two questions so important in this section?
4. Use the derivative of cosine and rules from Section 2.4 or 2.5 to differentiate ¹⁄_cos(x).

Submit answers through OnCourse

Reminder:

• Begin WW 6 and PS 6. Note that because of October break, this WeBWork will be due on Thursday, and the problem set will be due on Friday.
• Be sure to meet frequently with your group to work on your project -- you will find that even after figuring out the mathematics of your response, writing the response is difficult and takes time and much consultation. The calculations should be done by Wednesday at the latest.

Due Monday 10/10 at 8am

Enjoy October Break!

Due Wednesday 10/12 at 8am

Section 2.6 and Section 2.7

To read: Review both sections.

No Reading Questions Today

Reminder:

• Just a mid-semester reminder: some students feel that reading assignments are "busy work", but I have many reasons for assigning them (none of which are just to keep you busy): to ensure you have multiple exposures to the material, which helps it sink in; to teach you how to actively read technical material, by example; and to give you credit for the effort you put into reading -- "free points", since I don't grade on correctness.
• Bring your remaining questions on PS 6 to lab on Thursday.
• Continue working with your group on the project. Re-read the letter you received and make sure you've answered all the questions you were asked, however general or vague they might seem. Read the guidelines and the checklist before beginning to write your letter. It will not be easy to write, so leave plenty of time.
• We will be skipping Section 2.8.

Due Friday 10/14 at 8am

Section 2.9: The Mean Value Theorem

To read: All. As the text suggests, be sure to try drawing functions where f is continuous on [a,b], differentiable on (a,b), and f(a)=f(b), but doesn't have a horizontal tangent anywhere on (a,b).

Reading questions:

1. Suppose f(x) is continuous on [a,b] and differentiable on (a,b). Does Rolle's Theorem tell you how to find the point c in (a,b) such that f '(c)=0?
2. Why do we say that Rolle's Theorem is a special case of the Mean Value Theorem?
3. In the proof of Theorem 2.9.5, why does showing that f(b)=f(a) for the arbitrary points a and b allow us to conclude that f is constant on the interval I?

Submit answers through OnCourse

Reminder:

• If you are redoing Exam 1 for extra credit, remember it will be due Wednesday 10/19 at 2pm.
• There will be a differentiation exam during the last 20-25 minutes of class. Practice as wide a variety of differentiation problems as you can.
• PS 7 is another group assignment. Once again, I urge you to switch partners. If you were not the primary author last time, you should be this time. As always, do not split up the problems between you.
• Put plenty of time and thought into writing the final draft of the projects, which is due Monday at 5pm

Due Monday 10/17 at 8am

Introduction to Chapter 3
Section 3.2: Indeterminate Forms and l'Hôpital's Rule

To read: All

Reading questions:

1. Does l'Hôpital's Rule apply to lim_{x→ ∞} ^x2⁄_e^x ? Why or why not?
2. Does l'Hôpital's Rule apply to lim _{x→ ∞} ^x2⁄ _sin(x) ? Why or why not?

Submit answers through OnCourse

Reminder:

• If you need to re-take the differentiation exam, please do not put it off. And definitely do not put it off out of any sense of embarrassment or conviction that you don't want to come by my office until you can differentiate better -- many people successfully use the differentiation exam as an opportunity to ask, practice, and improve. The deadline for receiving 100% on the differentiation exam is Monday 10/24.
• The project has been extended -- now due Friday at 3:30 pm.
• We are skipping Section 3.1; instead, at the end of Chapter 3, we will be doing a section outside of your book on Taylor polynomials that covers similar but even more useful ideas.

Due Wednesday 10/19 at 8am

No Reading For Today (but there is for Thursday - see below)

No Reading Questions Today (but there are for Thursday)

Reminders:

• Exam 1 Rewrites are due at 2pm Wednesday, from those people who are doing it.
• Put plenty of time and thought into writing the final draft of the projects, which is due Monday at 5pm
• Exam 2 will be on Thursday 10/27.
• Bring your remaining questions on PS 7 to class.
• Remember to put a star next to the primary author's name.
• If necessary, keep taking the differentiation exam.

Due Thursday 10/20 at 8am

Section 3.3: Maximum and Minimum Values

To read: All

Reading questions:

1. For each of the intervals below, please answer the following question: If f is continuous on the interval, must f have an absolute maximum and an absolute minimum on that interval? If not, can it have an absolute maximum or an absolute minimum on that interval?
   1. [-5,5]
   2. (-∞, ∞)
2. Does the Extreme Value Theorem help with finding absolute extrema, when it applies?
3. Suppose that c is a critical number for f. Must f have a local extremum at x=c?

Reminders:

• Put plenty of time and thought into writing the final draft of the projects, which is due Monday at 5pm
• In order to receive 100% on the differentiation exam, you must pass it by 5pm on Monday 10/24.
• Exam 2 will be on Thursday 10/27.
• Bring your remaining questions on PS 7 to class Wednesday.
• Remember to put a star next to the primary author's name.

Due Friday 10/21 at 8am

Section 3.4: Increasing and Decreasing Functions

To read: All. As always, pay attention to the marginal graphs, comments, and charts -- most people find the charts that help determine where a function is increasing and decreasing to be very helpful. Also play close attention to the last paragraph in Example 3.4.1, as well as the paragraph in between Example 3.4.1 and 3.4.2.

Reading questions:

1. The graph of a function f '(x) from x=-10 to x=10 is shown below. Estimate the intervals on which f is increasing. How about decreasing?
   
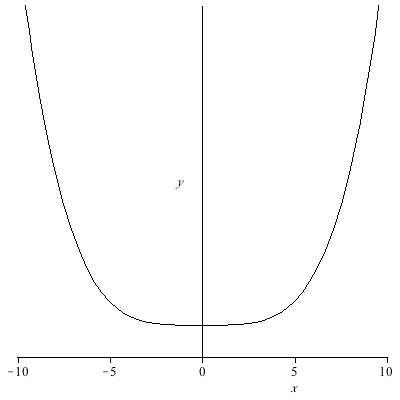
2. Use the Calculus methods from this section to determine on what intervals the function g(x), where g(x) is given below, is increasing and decreasing. g(x)=6x⁴-3x²+5000

Submit answers through OnCourse

Reminders:

• The project is due Friday at 2:30 pm.
• In order to receive 100% on the differentiation exam, you must pass it by 5pm on Monday 10/24.
• Get an early start on WW8 --as before, the study guide assumes you've completed the work on WW8.

Due Monday 10/24 at 8am

Section 3.5: Concavity and the Second Derivative Test

To read: All. Once again, and as usual, pay attention to all marginal comments, graphs, and charts. The charts for keeping track of concavity and inflection points are just as useful as those for determining direction and extrema.

Reading questions:

1. On page 287, consider Figures 3.5.55 a and b, and 3.5.56 a and b. In figure 3.5.55a, the graph is getting steeper, while in Figure 3.5.56a, the graph is getting flatter -- yet in both cases, the authors write that the slopes of the tangent lines are increasing. Similarly, in Figure 3.5.55b, the graph is getting flatter, while in Figure 3.5.56b, the graph is getting steeper -- yet in both cases, the authors say that the slopes of the tangent lines are decreasing. What is going on here?
2. Are all points where f ''=0 or is undefined automatically inflection points?

Reminders:

• Monday is the deadline for receiving full credit on the Differentiation Exam.
• As usual, put plenty of time and effort into studying for Exam 2; spreading your studying time out over several days is harder for some to plan, but much more effective than packing it into one or two days.

Due Wednesday 10/26 at 8am

Bring Questions for Exam 2

Wheaton's Honor Code
Wheaton's Description of Plagiarism
course policies

To read: Once again, as a reminder of how important the Honor Code is at Wheaton, I ask you to re-read the Honor Code, Wheaton's description of plagiarism, and the portion in the course policies that applies to the Honor Code, and to pay particular attention to how all of this applies to exam situations.

No Reading Questions Today

Reminder:

• As usual, take advantage of all the Kollett Center has to offer, as well as my office hours.
• Get questions on WW8 out of the way before class as much as possible, so you can be focusing on reviewing everything for the exam.
• Once again, you may have a "cheat sheet", consisting of notes handwritten by you on one side of an 8 1/2 x 11 (or smaller) piece of paper.
• You may begin taking the exam at 12:30pm Thursday.
• If you have not yet passed the Differentiation Exam, the next deadline is Monday 10/31 at 5pm, for 90% -- study for Exam 2 now, then get back to working on the Differentiation Exam.

Due Friday 10/28 at 8am

No Reading For Today

No Reading Questions Today

Reminder:

• As usual, get started on the next homework assignments
• For those who are still working on the Differentiation Exam, the deadline for receiving 90% is Monday 10/31 at 5pm.

Due Monday 10/31 at 8am

Section 3.6: Overview of Curve-Sketching

To read: All

Reading questions:

1. What is the point of learning how to sketch curves, given how available graphing tecnology now is?
2. In Example 3.6.4,
   1. How do the authors conclude that f'(x)<0 for all x ≠ a, -1?
   2. The authors say we can disregard the factor of x² +3x+3 in the numerator of f ''(x) because it is always positive. What is one way that you can figure out that it is always positive?
   3. Why does the factor x²+3x+3 being always positive mean that we can disregard it when discussing the sign of f ''(x)?

Submit answers through OnCourse

Reminder:

• The deadline for receiving 90% on the Differentiation Exam is Monday at 5:00pm.