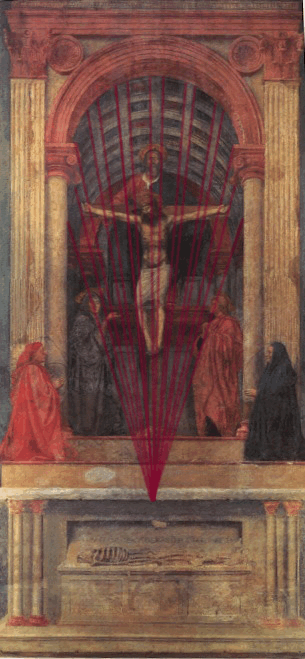
Lines that are perpendicular, or orthogonal, to the picture plane are called orthogonals.
Vanishing Point Theorem, Part 1:
If two or more lines in the real world extend away from the viewer so that they are orthogonal to the picture plane, then their perspective images have the same vanishing point.
That is, the perspective images of these lines are not parallel, and -- if fully extended -- their image lines will all intersect at the same point. That intersection is the vanishing point: no such line should extend beyond it.
In this drawing, we see (among other things) Jesus crucified in a barrel vault.
Whether or not you've ever actually seen a barrel vault in real life, you probably feel comfortable assuming that the beams in the ceiling of the vault that extend back are all parallel to each other, and are perpendicular to the front of the vault (which in turn looks as if it's parallel to the picture plane -- more on this shortly).
In short, it looks as if the beams are orthogonal to the picture plane.
From the Vanishing Point Theorem, Part 1 we know that if the painting is drawn in rigorous perspective, then when we extend these lines, they should all intersect in the same point -- the vanishing point --andthe viewer should stand directly opposite that point to properly fully appreciate the perspective.