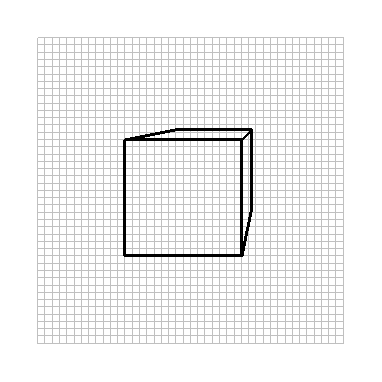
The box shown below represents a cube drawn in perfect perspective. We want to find the correct viewing distance (and the correct position for the viewer's eye) so that, when viewed from that spot, it looks like a cube.

| 1. Find the primary vanishing point. Since the front face of this box consists of two pairs of lines that still appear parallel in this drawing, we know that the front face is parallel to the picture plane. Since we know the box is a cube, we know that the sides are therefore perpendicular to the picture plane, and so the edge lines of those sides are orthogonals. That means that we know that this drawing is in true one-point perspective, and so we know that the viewer's eye should go opposite the primary vanishing point -- the vanishing point of the orthogonals. To find this primary vanishing point, which I have labeled V, I extend at least two of the orthogonal lines until they intersect. | 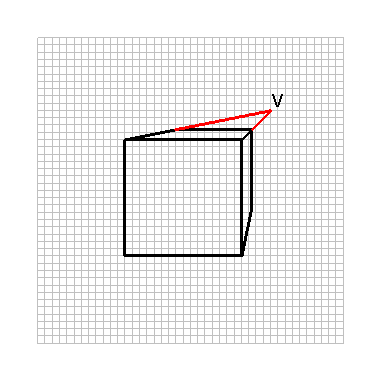 |
| 2. Draw in the horizon line (or the verizon line). I have drawn the horizon line -- the horizontal line through the vanishing point V. I could just as easily have drawn what we're calling the verizon line -- the vertical lines through V. |  |
| 3. Determine the correct viewing distance. Remember that in order to find the viewing distance, we look for a square either parallel to the "floor" or parallel to the "side wall". The viewing distance will be the distance between the primary vanishing point V and the vanishing point of the diagonal, V'. Because I have chosen to draw the horizon line, I need to look for a square that is parallel to the "floor", and then draw one of the two diagonals of that square. Had I chosen to work with the verizon line instead, I would need to look for a square that is parallel to the "side wall", and then draw one of those two diagonals. | 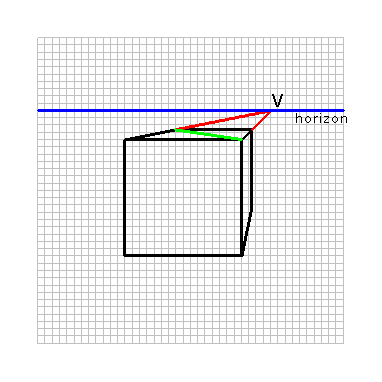 |
| Now I need to find the vanishing point of the diagonal that I have drawn. I'll call that the secondary vanishing point. Since my diagonal lies in a plane parallel to the "floor", its vanishing point will lie on the horizon line. I simply extend the diagonal until it hits the horizon line, and that intersection point will be the secondary vanishing point. (Had I been working with the diagonal of the side, it lies in a plane parallel to the floor, and so its vanishing point will lie on the verizon line.) The viewing distance is the distance between V and V'. | 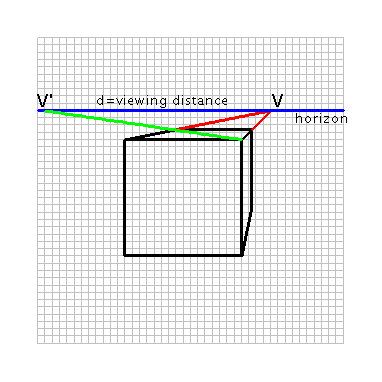 |
| 4. Determine the correct position for the viewer's eye. The viewer should place their eye directly opposite the primary vanishing point V, d units back from the drawing, where d is equal to the viewing distance we just calculated. |
Conclusion: Place your eye directly opposite the primary vanishing point; it should be the same distance back from it as the distance between the two vanishing points away.