Be sure to check back often, because assignments may change!
(Last modified: Thursday, January 26, 2012, 11:30 AM )

All section and page numbers refer to sections from Calculus: Early Transcendental Functions, 3rd Edition, by Smith and Minton.

I'll use WebWork and/or Maple syntax for some of the mathematical notation on this page. (Paying attention to how I type various expressions is a good way to absorb WebWork/Maple notation). I will not use it when I think it will make the questions too difficult to read.

Due Friday 9/2 at 8am

Course policies
Syllabus
Wheaton's Honor Code
Wheaton's Description of Plagiarism
Suggestions for reading a math text
Background questionnaire, on OnCourse
WeBWorK Orientation

To read: All of each of the above weblinks, except for WeBWorK Orientation. (See below for guidance on WeBWorK Orientation). Complete the questionnaire in OnCourse, if you're registered for the course already. (If you're not yet, complete it once you are).

As for WeBWorK Orientation: if you're registered, log in, use your Wheaton ID as your username and as your password (with a lower-case "w" in both cases). If you're not registered yet but did come to class Thursday and gave me your Wheaton ID, you should be able to log in using this same method by late afternoon Thursday. Otherwise, e-mail me with your name and Wheaton ID and I'll set up a WeBWorK account for you; in the meantime you can get started by using "guest" as both your username and password. The server may be slow, so be patient.

Once you're logged in, click on WeBWorK Orientation. Read the discussion on the right, and then click on Problem 1 to get started. Get as far as you can with the orientation tonight; we'll be working with WeBWorK in class on Friday -- those who just do a bit with orientation beforehand will continue working on it in class; those who finish it can begin reviewing precalculus material by working on the first WeBWorK assignment, WW 1 -- but be sure to finish WeBWorK Orientation first, as it's due Tuesday.

If you have trouble logging in to WeBWorK, e-mail me . Let me know exactly what you used for username and password -- cutting and pasting is the most exact way.

Reading questions:

1. Do you have any questions on the course policies, reading assignments, or syllabus? If so, what are they?
2. If a classmate, tutor, or friend not in the class helps you with your homework, should you acknowledge that help on your homework?
3. For a group homework assignment or a project, why is it unacceptable to divide the work between you, so that you each are only working on a portion of the assignment?
4. If you agree to be in a group with another person, but then do not fully participate in working through the homework problems or the project, why is it unacceptable to receive credit for that assignment?

Submit answers through OnCourse

Reminders:

• In these reading assignments, you should always briefly explain how you arrived at your answers.
• If you have a laptop and are comfortable bringing it to class, that would be helpful.
• By next Wednesday, you should have reviewed all of Sections 0.1 through 0.6, so get started on it now.
• The homework assignments are accessed through a link toward the bottom of the course's main web page.
• Begin WeBWorK Orientation, WW 1, and PS 1.

Due Wednesday 9/7 at 8am

A Description of Calculus 1 Homework Assignments
Problem Set Guidelines
Introduction to Chapter 0: Preliminaries
Section 0.1: Polynomials and Rational Functions
Section 0.2: Graphing Calculators and Computer Algebra Systems
Section 0.3: Inverse Functions
Section 0.4: Trigonometric Functions
Section 0.5: Exponential and Logarithmic Functions
Section 0.6: Transformations of Functions

Note:If this seems like a lot of reading, don't worry -- it's just this once, and most of it should be review for you. (Also, you've had nearly a week.)

To read: All of the Problem Set Guidelines. As for the introduction and the first six sections of Chapter 0, you may skip the portion of Section 0.4 on Inverse Trigonometric Functions and the portion of Section 0.5 on Hyperbolic Functions - we will leave them for Calculus 2 and Multivariable Calculus, respectively. Skim where the material seems very familiar, read more carefully where it seems new or to be presented in a different way than you are used to. Don't worry if you are not especially comfortable with some of it (especially logarithms), as long as you've seen it before. If large chunks of the material are completely unfamiliar to you, please come see me as soon as possible.

In Section 0.3, pay particular attention to the idea of one function reversing the action, or "undoing", another. Also pay attention to the distinction between the notations f ^-1(x) and [f(x)] ^-1.

In Section 0.4, make note of the table of common values of sine and cosine as they are handy to know. If these values do not look familiar to you, please come see me as soon as possible to discuss why they're true and for tips on how to remember them.

Reading questions:

1. Below is a graph of the function f(x)=x⁴ +  ^x3⁄₂ - ^5x2⁄₂ + x over the interval [-1,2].
   
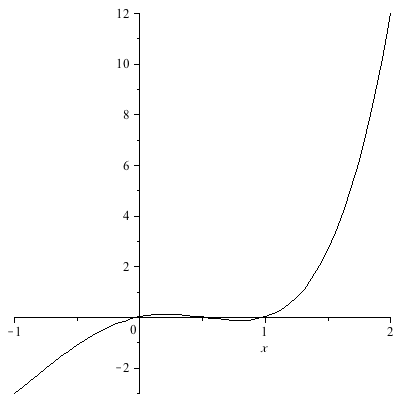
   1. What intervals for x and y satisfactorily display all the x-intercepts and local extrema of this function? (Feel free to use a graphing calculator, the on-line program Wolfram Alpha, or Maple, the Computer Algebra System Wheaton uses, which is available in Mars Science 1141, the computer lab, and many of the computers in the Kollett Center.)
   2. From your graph, estimate the locations of all local minima.
   3. From your graph, estimate the locations of all x-intercepts.
   4. From your graph, estimate the locations of all inflection points.
2. Can a function exist, even if you are unable to write down a precise formula for it? Give an example of how such a function might arise.
3. 1. What is the radian measure of an angle?
   2. What is 1^o, in radians?
   3. In Calculus, what unit of measure do we always use for angles?
4. How are the functions f(x)=3^x and g(x)=log₃(x) related?
5. Let f(x)=x+1 and g(x)=e^x.
   1. What is the composition f o g ?
   2. What is the composition g o f ?
6. Let g(x)=2x³.
   1. How is the graph of h(x)=g(x)-5 related to the graph of g(x)? Why?
   2. How is the graph of j(x)=g(x-5) related to the graph of g(x)? Why?

Submit answers through OnCourse

Reminders:

• WeBWorK orientation is due Tuesday at 5pm.
• WW1 is due Thursday at 1pm and PS 1 is due Friday at 11:30am. WeBWorK assignments are always to be done individually; although problem sets will alternate between individual and group assignments, PS 1 is an individual assignment. Read and follow the problem set guidelines referred to above.
• As mentioned in the Course Policies, in order to begin to determine whether you have any gaps in your background, I have prepared a Preliminary Assessments, that I've broken up into four WebWork files. These are listed on WebWork in the same place as the WebWork assignments, but they are below all the usual webwork assignments. Begin working on these -- you need to have made a legitimate attempt at each question on each assessment by Monday 9/12. (Be aware that unlike the usual WebWork assignments, these assessments each have a time-limit.) You have until Monay 9/12 to get 100% on each -- the idea is that if you're rusty on any topic, you'll come to my office hours and we can decide jointly how you should get up to speed.
• If you're having difficulties with the homework, or if you'd just like to make sure you're on the right track, come to my office hours and/or visit the Kollett Center during the Calc tutoring hours.
• If you have any remaining questions on the problem set after coming to my office hours and visiting the Kollett Center, bring them to class Wednesday - I will spend roughly 10 minutes at the beginning of class giving hints to the most common student questions.
• Make sure you've read all the introductory material on the course web page.

Due Monday 9/12 at 8am

Section 1.2: The Concept of Limit

Section 1.4: Continuity and Its Consequences

To read: All of Section 1.2. Parts of Section 1.4 fit in very naturally with the ideas of Section 1.2, so read the beginning of Section 1.4, through Example 1.4.3 (top of page 100) also.

Note: Again, with losing the first day of class as we did, we may not or may not get to these sections until Wednesday, but reading some for Monday is better than reading three sections for Wednesday.

Reading questions:

1. Consider the graphs of f(x)=^(x2-4)⁄_(x-2) and g(x)=^(x2-5)⁄_(x-2) , the two functions that the text discusses in the opening of Section 1.2.
   1. Discuss the similarities and the differences between the two graphs.
   2. If we think of what's happening at x=2 in each graph as a problem, for which function can that problem be fixed, and how (graphically)?
2. In Example 1.2.2, the authors cancel the common term of x+3 from both the numerator and the denominator, which takes a fraction which does not exist at x=-3 and turns it into one that does. And yet, a function either exists at a point or it doesn't. Why do you think it is "legal" for the authors to cancel the common term of x+3?
3. When figuring out a limit by either looking at a graph or by creating a table of nearby values, can you conclude that you know that limit for sure?
4. Let f be a function.
   1. True or false (and why): If f(a) exists, f must be continuous at x=a.
   2. True or false (and why): If lim_{x → a} f(x) exists, then f must be continuous at x=a.

Submit answers through OnCourse

Reminder:

• You should have made at least a first attempt at each of the four Preliminary Assessments by Monday morning at 8am. Any of these that you have already received 100% on, you are finished with, and we can feel reasonably comfortable that any hole in that area you might have is relatively small. Continue to work on those that you have not yet received 100% on; the deadline for successfully completing each one is Monday 9/19. If you are having trouble, please do come to my office hours as soon as possible.
• Office Hours and/or tutoring hours are an important part of Calculus for all students; do not hesitate to take advantage of them.
• Just a reminder: the point of reading assignments is partially to ensure you do the reading, because multiple exposures to the material helps it sink in, partially to teach you by example how to actively read technical material (which includes asking yourself questions), and partially to give you credit for reading. I do not expect you to have mastered the section by the time you answer these questions, and do not grade on the correctness or lack thereof of your answers, only on whether your answers indicate a genuine attempt. Use this as an opportunity to identify the material you find particularly mysterious before class.

Due Wednesday 9/14 at 8am

Section 1.3: Computation of Limits
Section 1.4: Continuity and its Consequences

To read: All of Section 1.3, except skip the portions of Theorem 3.4 that relate to inverse Trigonometric Functions and skip Example 1.3.6. Finish Section 1.4. In the discussion of the tax rate schedule that precedes Example 1.4.8, work to understand where the piecewise-defined function comes from, and then be sure to understand Example 1.4.8 itself.

Reading questions:

1. Apply the rules of limits to evaluate the following:
   1. lim_{x → 5} ^(x2-2x+7)⁄_(x-3)
   2. lim_{x→ -3}^(x2+x-6)⁄_(-x-3)
   3. lim_{x→ 1} (e^2x-1) ^1/2
2. In Example 1.3.8, what role did looking at a graph and a table of values play, and what role did using the Squeeze Theorem play?
3. When a function f is continuous at x=a, how can you compute lim_{x →a} f(x)? Why should you not use this technique when the function is discontinuous at x=a?
4. In Example 1.4.2, if we define g(1)=7, is g(x) continuous at x=1? Why or why not?
5. Why are the discontinuities in Figure 1.22b and Figure 1.22d not removable?
6. Without looking at a graph, how can we conclude that f(x)=e^cos(x)-1 has a zero on the interval [0, π]?

Submit answers through OnCourse

Reminders:

• You are aiming to have received 100% on each of the assessments by Monday 9/19. If you've been giving it every effort but are having trouble, please come talk to me.
• Your group will turn in one joint version of PS 2; the recopying should all be done by one person, the "primary author". Put a star next to the name of the "primary author".
• If, after coming to office hours, you still have questions on PS 2, bring them to class on Wednesday.
• Be sure that each member of your group has a photocopy of the problem set you turn in, both for studying purposes and for your records.
• I suggest that you switch partners with every group problem set. You should be the primary author roughly every other group problem set.

Due Friday 9/16 at 8am

Section 1.4: Continuity and its Consequences

To read: All. Pay particular attention to the Intermediate Value Theorem and how it can be used.

No Reading Questions Today
Reminders:

• Continue to work on any of the four Preliminary Assessments you have not yet received 100% on. Remember that the point of these is two identify weaknesses in your background early on, so that we can work to address them before they get too much in your way. This only works if you give yourself the time to work on them, and if you come in for help. Also remember, they count as two homework assignments, once completed. The deadline is Monday 9/19.
• Begin WW3 and PS 3. PS 3 is an individual assignment.
• I just want to remind you again to take advantage of both my office hours and the Kollett Center!
• In this course, we will occasionally skip sections. We will be skipping Section 1.6, as I believe this material can wait until a later course (for those who continue on).

Due Monday 9/19 at 8am

Section 1.5: Limits Involving Infinity; Asymptotes
Section 1.7: Limits and Loss of Significance Errors

To read: All of Section 1.5, except for Example 1.5.10. As for Section 1.7, I'd just like you to skim through it, to get a feel for what can go wrong when relying on a computer or a calculator without understanding what it's doing.

Reading questions:

1. Does lim_{x → -3} ¹⁄_(x+3)⁴   exist? If so, what is it? If not, why not?
2. Discuss the left- and right-sided limits for lim_{x → 4} ^(x-1)(x-3)⁄_(x-6)(x-4).

Submit answers through OnCourse

• The deadline for the four Preliminary Assessments is Monday 9/19.

Due Wednesday 9/21 at 8am

Introduction to Chapter 2
Section 2.1 Tangent Lines and Velocity

To read: All

Reading questions:

1. Describe in your own words what a secant line and a tangent line to a curve is.
2. Can a tangent line intersect a curve at more than one point?
3. Let f(x)=x²+x.
   1. Find the slope of the secant line from x=1 to a nearby point x=1+h.
   2. For the above secant line, must the value of h be positive? (Explain)
4. Briefly explain the difference between speed and velocity.

Submit answers through OnCourse

Reminders:

• Bring remaining questions on PS 3 to class.
• Exam 1 will be Thursday 9/29.

Due Friday 9/23 at 8am

Section 2.2: The Derivative

To read: All. Be sure to pay particular attention to Examples 2.2.5 and 2.2.6--these examples are extremely important as they illustrate, much more than any formula can, what is really going on with derivatives.

Reading questions:

1. In the first paragraph of the section, the authors say that the fact that otherwise unrelated notions can be described by the same mathematical expression is an indication of the power of mathematics. Why do you think this is an indication of math's power?
2. Let f(x)=2x²-3x .
   1. Mimic Example 2.2.1 to compute the derivative of f(x) at x=1.
   2. Mimic Example 2.2.2 to compute the derivative of f(x) at an unspecified value of x. Use your result to compute the derivative of f(x) at x=1, x=2, and x=3.

      For those of you who have learned derivatives before: You may know a shorter way of doing this problem. Nonetheless, please proceed as in Example 2.2.1, to practice the ideas of the definition of the derivative.

3. In many of the examples in the text, and in the previous reading question, we find the derivative of a function at an unspecified point. What is the benefit of doing this?
4. The graph of a function g(x) is shown below.
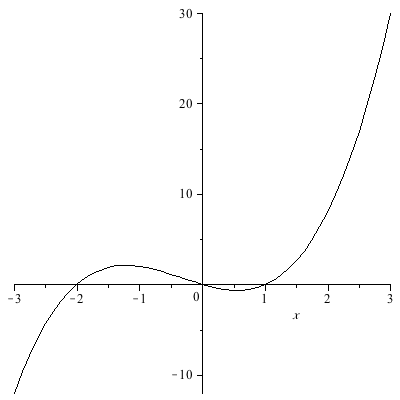
   1. On what intervals of x is the slope of the tangent line positive?
   2. On what intervals of x is the slope of the tangent line negative?

Reminder:

• Get an early start working on WW4. There is no PS 4 for this week, so that you can get instant feedback on all the homework before the exam. The questions on the study guide assume that you have already worked through WW4. (The study guide is not on WebWork, because you need to practice writing solutions up, as that's the format of the exam itself.)

Due Monday 9/26 at 8am

Section 2.3: The Power Rule

To read: All

Reading questions:

1. What is the slope of the line tangent to y=2x?
2. Let f(x)=-5x²+7x-4. Compute as many derivatives as possible, as in Example 3.6.
3. Let g(x)=⁵⁄_x². Compute g ', g '', g '''. Why am I not asking you to compute as many higher-order derivatives as possible, as in the previous question and Example 3.6?

Submit answers through OnCourse

Reminder:

• Spread your studying for the exam out over several days -- I know it's difficult for some people to study that way, but the material really does sink in better when you do.

Due Wednesday 9/28 at 8am

Bring Questions for Exam 1

Wheaton's Honor Code
Wheaton's Description of Plagiarism
Course policies

To read: Re-read the Honor Code, Wheaton's description of plagiarism, and the portion in the course policies that applies to the Honor Code. Pay particular attention to how all of this applies to exam situations.

No Reading Questions Today

Reminders:

• As you prepare for the exam, be sure to take advantage of all the Kollett Center has to offer, as well as my office hours!
• Get questions on WW4 out of the way before class! This problem set should be finished before class, so you can be focusing on reviewing the semester to date.
• For the exam, you may have a "cheat sheet", consisting of notes handwritten by you on one side of an 8 1/2 x 11 (or smaller) piece of paper.
• You may begin taking the exam at 12:30pm Thursday.

Due Friday 9/30 at 8am

Section 2.4: The Product and Quotient Rules
Section 2.5: The Chain Rule

To read: All of Section 2.4. In Section 2.5, read through Theorem 2.5.1 (the bottom of the first page).

Reading questions:

1. The second sentence of Section 2.4 says "...you might wonder whether the derivative of a product of two functions is the same as the product of the derivatives." Why might you think that?
   
2. Find the derivative of (³⁄_x² - √ x)(6x³+8x²-14).
3. Find the derivative of ^{(3⁄_x2 - √ x)} ⁄_{(6x³+8x²-14)}.
4. Consider the "simple examples" that open Section 2.5, on page 189.
   1. Based only upon those opening paragraphs, why would you suspect that the derivative of (x²+1)⁴ is 4(x²+1)³(2x)?
   2. Again in the spirit only of the opening paragraphs of the section: if as the book suggests toward the bottom of page 189, we let g(x)=x²+1 and f(x)=x⁴, then what should f '(x) be? f '[g(x)]? g'(x)? How are these pieces related to the conjectured derivative above?

Submit answers through OnCourse

Reminder:

• Begin WW 5 and PS 5. PS 5 is your second group assignment. Most people find that it's a really good idea to work with somebody different each time for the first several group problem sets, so I urge you to switch partners. If you were not the primary author last time, you should be this time. As always, do not split the problems up between you.