Be sure to check back often, because assignments may change!
(Last modified: Monday, February 11, 2008, 9:59 AM )

I'll use Maple syntax for mathematical notation on this page.
All section and page numbers refer to sections from Smith & Minton's Multivariable Calculus, 3rd edition.

Due Friday 1/25 at 9am

guidelines for submitting reading assignments
suggestions for reading a math text
course policies
syllabus

• To read: all
• Pay attention to: all of it. Any questions? Please do ask me!

Section 10.1: Vectors in the Plane
Section 10.2: Vectors in Space
Section 10.3: The Dot Product
Section 10.4: The Cross Product

• To read: All of sections 10.1, 10.2, and 10.3; up through page 818 in Section 10.4. Don't worry, you won't usually have this much to read!
  In this course, the graphs and diagrams are critical, and the authors really put a lot of effort into explaining them. Many people have a hard time plotting points on 3D axes (see section 10.2) -- really try to follow what the authors are describing in their diagrams!

E-mail Subject Line: Math 236 Your Name 1/25

Reading questions:

1. Find a unit vector in the direction of <-2,3,-6>.
2. Let a=<2,-1,3>, b=<4,10,-1>, and c=<3,-1,2> be vectors, and let x.y represent the dot product of any vectors x and y.
   

   (a) Find a.b.
   (b) Does (a.b).c make sense, and if so, what is it in this case?
   (c) Are b and c orthogonal?
   

3. Briefly explain what, geometrically, proj_ba and comp_ba are.
4. How is a x b related to a and b geometrically?
5. If a and b are vectors in V₂ (that is, in the plane), is a x b defined?

Reminders:

(I start out with extensive reminders at the beginning of the semester -- often things I'm afraid I'll forget to mention. I pare them down significantly as the semester goeos by.)

• In these assignments, you should always briefly explain how you arrived at your answers.
• Remember, if you have any questions, please come to my office hours. Office hours will probably be a huge part this class; I expect nearly every one will come see me at least every couple weeks.
  
• Begin Problem Set 1, listed at the bottom of this course's web page. . The problem sets due each Friday (except this first Friday, 1/25) reflect an entire week's worth of work, and you should be working on them throughout the week. PS 1, due 2/1, will actually reflect more than a week's worth of work, and as such will be unusually long, so plan on spending plenty of time on it!

Please Note:

You should receive an automated response letting you know I've received your reading assignment ... if your subject headings exactly match the filters I've set up. If you don't receive such a message, it might mean I didn't get your response, but it might also just mean that your subject heading didn't match my filters. If you don't receive a response, just check with me now and then to make sure I've gotten everything you've sent in. Sometimes messages do seem to just disappear, so (if your "sent-mail" box is emptied everynow and then, or if you're not sure about that) send yourself a copy of every reading assignment, just in case you need to re-send one to me.

Due Monday 1/28 at 9am

Problem Set Guidelines
Section 10.4: The Cross Product
Section 10.5: Lines and Planes in Space

• To read: Finish Section 10.4; read 10.5 thru page 830. You will notice throughout this book that there are many applications -- in section 10.4, you encounter torque and magnus force. We won't always be able to spend much time on the applications, but still read them to get a sense of the place of multivariable calculus in the world. Those of you with a related background (in this case, physics) will probably get more out of these applications than others; if the details of such examples prove stressful, focus on the big picture.

E-mail Subject Line: Math 236 Your Name 1/28

Reading questions:

1. If a, b, and c are arbitrary vectors, should we expect that (a x b) x c=a x (b x c)?
2. How can we tell whether two vectors in V₃ are parallel? How can we tell whether two vectors are orthogonal?
3. What information about a line L do you need to determine an equation for the line?
4. Find parametric equations for the line through the point (1,2,3) and parallel to the vector <3,0,-1>.

Reminder:

• For the problem sets, it really is a good idea if you work on the non-focus ("black") problems first -- I choose those to be good problems for helping understand the ideas and techniques. You'll find that writing up the focus ("red") problems goes much more smoothly if you've already worked through the non-focus problems.
• PS 1 is an individual assignment. While this of course means that you will each be turning in your own problem set, it does not mean that you can not work with someone else, as long as you do so in a way that helps you all better understand the material. This generally means that you each think about the problems on your own, and then discuss your ideas. It often will also mean working through the problems on your own, and then comparing results. When it comes time to write the problems up, you should each do so on your own. Just to be clear: You absolutely may not divide the problems up between you, nor may you copy somebody else's work, merely changing a word or two (see the section on the Honor Code in the Course Policies).

• Make sure you've read all the stuff I handed out in class and all the material on the course web page.

Due Wednesday 1/30 at 9am

Section 9.1: Plane Curves and Parametric Equations Section 10.5: Lines and Planes in Space

• To read: Finish Section 10.5. Assuming I remember to hand out Section 9.1, read through it to help you put parametric equations of lines into a larger context. After reading it, skim through the first portion of Section 10.5 again.

E-mail Subject Line: Math 236 Your Name 1/30

Reading questions:

1. Find parametric equations for the line through the points (1,2,3) and (7,-1,0).
2. What information about a plane P do you need to determine an equation for the plane?
3. Find an equation for the plane containing the point (1,2,3) with normal vector <3,0,-1>.

Reminder:

• Make sure you clear up any difficulties on PS 1 by coming to my office hours or taking advantage of the tutors at the Kollett Center.

Due Friday 2/1 at 9am

Section 11.1: Vector-Valued Functions

• To read: Everything but Example 1.8. Really think about Example 1.5 -- before you ever turn the page, can you make some progress on figuring out which vector-valued functions do (or do not) go with which graphs? When the book refers to past sections that you haven't studied (for instance, curves defined parametrically) don't panic -- just figure out as much as you can about it, and move on.

E-mail Subject Line: Math 236 Your Name 2/1

Reading questions:

1. The vector-valued function r(t)=cos(t)i+sin(t)j lies in the plane.

   (a) Using Example 1.2 as a guide, what will the graph of this function look like?
   (b) Is it possible to rewrite r(t) as a function y=f(x)?

2. What are some advantages to using vector-valued functions?

Reminders:

• PS 1 is due at the beginning of class Friday.
• Quick reminder: the problem sets due each Friday reflect the whole week's worth of work, and should be worked on throughout the week, so plan on starting PS 2 this week-end. As always, start with the non-focus problems. It's a group problem set, so find a partner to work with now, to work with after you've given some thought to the problems. (You must work in a group. Groups of 2 are best; 3 is less than ideal, but sometimes unavoidable. I do not want you to turn in a hw you've done on your own, neither do I want you to work in a group of 4.) As always, don't split the problems up between you.

Due Monday 2/4 at 9am

Section 11.2: The Calculus of Vector-Valued Functions

• To read: All.

E-mail Subject Line: Math 236 Your Name 2/4

Reading questions:

1. If r(t)=tcos(t)i+exp(t^2)j+ln(t)k, what is r'(t)?
   Remember exp(t^2) is Maple notation for e^t2.
2. If r(t) is a vector-valued function, what geometric/graphical information does r'(a) give you?
3. Let r(t)=cos(t)i+sin(t)j and s(t)=sin(5t)i+cos(5t)j.

   (a) What do the graphs of r(t) and s(t) look like?
   (b) If the graphs of two vector-valued functions r(t) and s(t) are the same, must r'(0)=s'(0)? (Is this a new result, or was it also true for functions f(x) and g(x)?)

Reminders:

• Check out the tutoring hours for Multi at the Kollett Center office hours held by student tutors.

Due Wednesday 2/6 at 9am

Problem Set Guidelines
Section 11.3: Motion in Space

• To read: All. Pay particular attention to Examples 3.4 and 3.7. We probably won't spend much time on rotational motion, but still skim through those examples (3.3, 3.5, and 3.6).

E-mail Subject Line: Math 236 Your Name 2/6

Reading questions:

1. If r(t) is a vector-valued function representing the motion of an object at time t, what physical information does r'(t) give you? How about ||r'(t)||?
2. Find the velocity and acceleration vectors, if the position of an object moving in space is given by r(t)=(5/sqrt(t))i+ln(t³)j-tan(3t)k.

Reminders:

• For the group problem sets, each group will turn in one joint problem set. You should work together to solve every problem, then one of you should take responsibility for writing up the entire problem set. That person is the "primary author".
• These groups are not permanent -- you'll be switching partners from week to week. You should end up being primary author 1/3 - 1/2 the time (depending on whether you're working in groups of 2 or 3).

Due Friday 2/8 at 9am

Section 11.4: Curvature

• To read: All

E-mail Subject Line: Math 236 Your Name 2/8

Reading questions:

1. Explain the idea of curvature in your own words.
2. If the helix in Example 4.5 were changed to r(t)=< 2sin(t), 2cos(t), 4t²>, will the curvature still be constant? Don't actually do the calculation, but give an intuitive justification - think about how changing the z-coordinate to 4t² from 4t will affect the graph.

Reminders:

• Make sure each member of your group has a photocopy of the problem set (for studying purposes). When you turn in the problem set, put a star next to the primary author's name.

Due Monday 2/11 at 9am

Section 11.5: Tangent and Normal Vectors

• To read: Read through example 5.2, then skip to the section on Tangential and Normal Components of Acceleration. When reading about Tangential and Normal Components of Acceleration, don't stress too much about the details - focus mainly on the big picture and on the results.

E-mail Subject Line: Math 236 Your Name 2/11

Reading questions:

1. Suppose you are skiing down a hill along a path that curves left. Describe the direction of the unit tangent and principle unit normal vectors to the curve that describes your motion.

Reminders:

• Just a reminder that you really will find the focus problems easier if you work through the non-focus problems first.

Due Wednesday 2/13 at 9am

Section 10.6: Surfaces in Space

• To read: All. If possible, read while sitting by a computer with Maple, and try doing their examples in Maple, using the Maple "cheat" sheet I handed out in class. If it's not working for you, though, don't waste hours on it -- instead come talk to me about Maple soon!

E-mail Subject Line: Math 236 Your Name 2/13

Reading questions:
Consider the surface x=4y²+4z².

1. Which coordinate plane does the equation z=0 define?
2. What does the trace of this surface in the xz-plane look like?
3. What do the traces of this surface in the planes x=k look like?
4. What is this quadric surface called?

Reminders:

• Once again, remember that while it's okay to consult with others on the big ideas behind problems, you get alot more out of it if you think through the problems as much as you can before and after such consultations. Also, remember you must write up the problems on your own, in your own words. If you're having any difficulties at all, please do come to my office hours and/or go to the tutors at the Kollett Center.

Due Friday 2/15 at 9am

Section 10.6: Surfaces in Space

• To read: Re-read Section 10.6 Again, use Maple while you're reading and really get a feel for the different surfaces. Pay particular attention to the table at the end of the section.

No Reading Questions Today

Due Monday 2/18 at 9am

Section 11.6: Parametric Surfaces

• To read: All. I'd again suggest reading while sitting by a computer with Maple, so that you can follow along with their comments about what things look like when graphed various ways, with the help of your cheat sheet. And again, if you don't feel like you know enough Maple to do this, please come see me!
  When you get to the discussion before Example 6.4, don't panic that you haven't seen polar coordinates much: all you need to know is what they tell you.

E-mail Subject Line: Math 236 Your Name 2/18

Reading questions:

1. Following a process similar to that in Example 1 (not using Maple to graph), identify the surface defined by the parametric equations x=2cos(u)sin(v), y=2sin(u)sin(v), z=6cos(v). (You might want to review the generic equations for quadric surfaces given in Section 10.6).
2. In Example 6.3, what might make you think to use cosh(u) and sinh(u) in the parametric equations for x and y? That is, what property that's useful in this situation do they have?

Due Wednesday 2/20 at 9am

Section 12.1: Functions of Several Variables

• To read: Up through middle of page 925. This is another section where reading where you have access to Maple would not be a bad idea. When you get to example 1.6, try to figure out as much as you can about which functions go with which graphs before you read the solution; when you do read the solution, do so extremely carefully.

E-mail Subject Line: Math 236 Your Name 2/20

Reading questions:

1. Below are the graphs of 4 surfaces. 2 of them are the graphs of the functions f₁(x,y)=[(x²+y²)-2]³ and f₂(x,y)=y²sin(x). Match the functions with their graphs.

   (a)	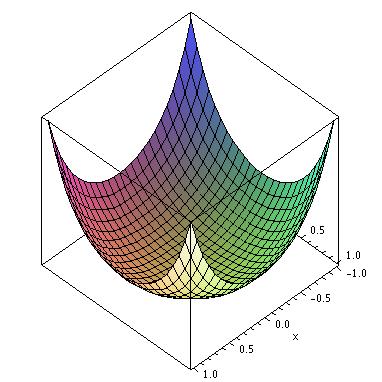	(b)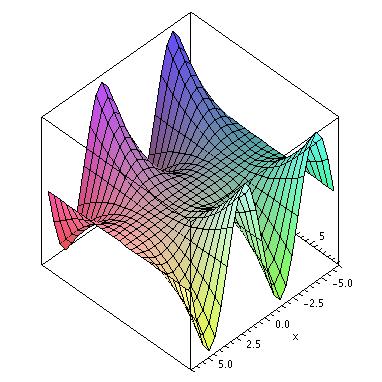
   (c)	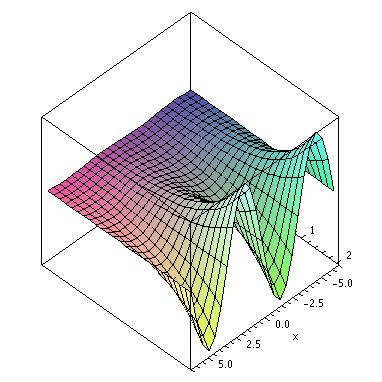	(d)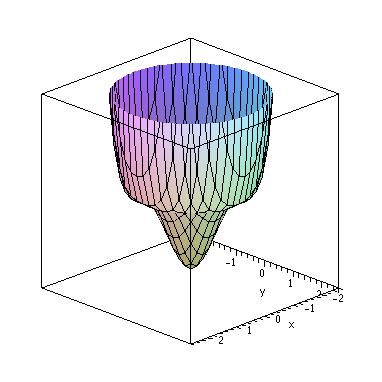

Reminders:

• The problem set due Friday is a group problem set. Work with a different partner this time! As usual, don't divide the problems up between you. If possible, the primary author this time around should not have been the primary author last time.
• The in-class portion of Exam 1 will be Wednesday 2/27. You will receive the take-home portion that day, and return it Monday 3/3.

Due Friday 2/22 at 9am

Section 12.1: Functions of Several Variables (continued)

• To read: Finish. Pay particular attention to the definition of level curves and contour plots.

E-mail Subject Line: Math 236 Your Name 2/22

Reading questions:

1. Give the equations of two level curves for the function f(x,y)=x²/4+y²/9. What will these level curves look like?
2. Below is a contourplot for the function sin^2(x)+cos^2(y). Roughly where does it have local extrema? How can you tell?
   
   
   (I used the Maple command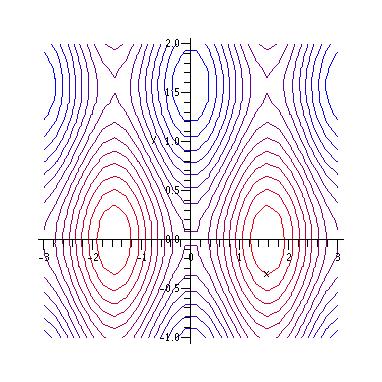
   contourplot((sin(x))^2+(cos(y))^2,x=-3..3, y=-1..2, contours=15, coloring=[blue,red]);
   to create this graph. The "coloring=" option allows you to specify which color will be associated with the lowest contours (blue) and which will be associated with the highest (red). )

Reminders:

• Make sure each member of your group has a copy of the problem set. Put a star next to the primary author's name.

Monday 2/25 at 9am

Section 12.2: Limits and Continuity

• To read: All. Many (most?) have not seen the formal definition of limit (the epsilon-delta definition) for functions of one variable. Don't worry about it. Focus on understanding the idea behind the epsilons and deltas, and what it means graphically.

E-mail Subject Line: Math 236 Your Name 2/25

Reading questions:

1. Consider f(x,y)=3x² /(x²+y²). We want to see what we can say about lim_{(x,y) -> (0,0)} f(x,y).

   (a) Find the limit along the path x=0. That is, find lim_(0,y)->(0,0) f(0,y).
   (b) Find the limit along the path y=0. That is, find lim_{(x,0) -> (0,0)} f(x,0).
   (c) What -- if anything -- can you conclude from your results?

2. What is the point of Example 2.5?
3. Why do you suppose we're studying limits now?

Reminders:

• Please come clear up any lingering difficulties before the test Wednesday.

Due Wednesday 2/27 at 9am

Exam 1 (in-class portion)- No new reading!

Due Friday 2/29 at 9am

Section 12.3: Partial Derivatives

• To read: All

E-mail Subject Line: Math 236 Your Name 2/29

Reading questions:

1. For a function z=f(x,y), what information does f_y(1,2) give you?
2. For f(x,y)=ye^xy, find f_x(x,y).
3. How many second-order partial derivatives does a function f(x,y,z) have?